Unraveling the power of real quantum computers
We work on the identification, quantification and operational use of quantum resources, such as quantum coherence and quantum correlations, for information processing in realistic scenarios (open quantum systems that are subject to noise). We conceive analytical and computational methods to detect and quantify elusive properties of quantum systems, such as quantum superposition (coherence) and various forms of quantum correlations. We study how to harness genuinely quantum cooperative effects between quantum particles as a resource for quantum computing, artificial intelligence, metrology and communication. Moreover, we investigate how to efficiently extract information from quantum machines. A register of a few qubits is a very complex system: the complete description of the quantum state (tomography) of ten atoms requires about one million measurements! We concoct experimental strategies to evaluate key properties of many-body quantum systems, e.g. coherence and entanglement, without performing expensive state tomography. Our findings help evaluate the true computational power of quantum computers.
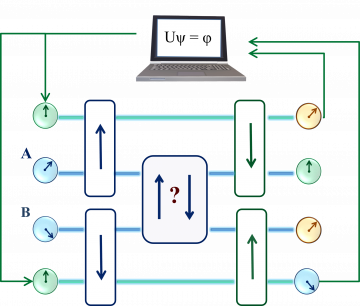
X. Yuan, Q. Zhao, D. Girolami and X. Ma, Quantum Coherence and Intrinsic Randomness, Adv. Quant. Tech. 2, 1900053 (2019)
B. Yadin, J. Ma, D. Girolami, M. Gu and V. Vedral, Quantum processes which do not use coherence Phys. Rev. X 6, 041028 (2016)
J. Ma, B. Yadin, D. Girolami, V. Vedral and M. Gu, Converting Coherence to Quantum Correlations, Phys. Rev. Lett. 116, 160407 (2016)
D. Girolami, Observable measure of quantum coherence in finite dimensional systems, Phys. Rev. Lett. 113, 170401 (2014)
